Separating runners in Orienteering: Part III
It is time to dig into the extensive report on separating runners in orienteering again. This time we take a look at the different separating methods discussed in the report – and their applicability to a long distance race with a high quality start field. We also take a look at the runners comments to the different separating methods – the fairness issue is given special focus in this regard.
For background information about the report, see part I which gives a general overview of the report along with main conclusions.
Start Interval
The second article related to the report on separating runners in Orienteering, part II, considered the start interval for World Orienteering Championships long distance. It was interesting to see that only 7% of the 460 voters wanted to keep the start interval for WOC long distance as short as it is today (2 minutes) or make it shorter (1 minute). A large majority – more than 80% – want a longer start interval (at least for the red group), whereas as much as 10% of the voters want to have a mass start instead of individual start for WOC long distance. Out of interest, I’d like to check if the percentage is even higher than 10% when the question is posed more directly:
What went wrong in previous Championships?
Map from WOC Long distance 2005, Japan
The report has an interesting section about what went wrong in previous Championships. This gives a good background for discussing separating methods. The complete section is included unabridged below:
In some World class events there has been large groups forming. WOC 1993, 1997 and 2005, WC Switzerland 1996 and Sweden 2002 comes to mind. Is it possible to find a common theme?
- We have found that groups mostly form when a (weaker) runner makes a mistake (near the control) and is overtaken by a stronger runner. We would thus expect any system that mixes weaker runners (which are more likely to make mistakes) with stronger runners (that make less mistakes) to prevent bunching. The World Cup races in Switzerland and Sweden were mass-start races so here the weaker and stronger runners obviously were mixed.
- WOC 1993 (USA) was without qualification races. This implies that every nation had the right to one runner in each start group. This also means that there were a few weaker orienteers started in the last start group as also the weaker nations could put one of their runners in this group. Maybe more important for the formation of large groups it also meant that the stronger nations had one of their runners in an early start group with many weaker runners. It has also been examples of races with qualification heats where one of the pre-race favourites makes a relatively large mistake in the qualification, and then acts as a broom sweeping through the field in the final. The women’s middle distance in WOC 2007 is one recent example. In the races mentioned above we would expect many of the groups to be H-groups [Editors note: See the report for definition of H-groups.]
- WOC 1997 (Norway) took place in extremely detailed and difficult terrain, with rather rough ground. The start interval was reduced to 2 minutes for the first time. There were qualification races over similar terrain, using the same map. It is difficult to measure the difficulty by objective means, but the qualification races seem simpler than the finals. For this championship there were also only two qualification heats with 30 from each heat to the final. The course for the final had many controls (24 for the men) and many short legs.
- WOC 2005 had the qualification race in a relatively simple terrain, while the organisers (like all other organisers) spared the most demanding terrain for the final. A simpler orienteering for the qualification race meant that some weaker runners could obtain a good start position in the finals. The final map contained many short legs in the beginning of the course.
Map from WOC 1993 Classic, USA
In WOC 2001 (Finland) and 2004 (Sweden) the finals took part in detailed terrains, but without many groups forming. Here the qualification races were in equally difficult terrain, thus the weaker runners were already sorted out. In WOC 2006 (Denmark) and WOC 2007 (Ukraine) both the qualifications and (long) finals were in fairly simple terrain. Again there were few groups forming.
It should also been noted that NOC (Nordic Open Orienteering Championships) which uses a fairly strict ranking system for the start list have had no problem with large groups forming.
Thus the main conclusions from previous “disasters” is that equal difficulty should be used for qualification and finals, there should be qualification races, and that the first part of the course should not contain many short legs with difficult orienteering.
Map from WOC 2004 Long distance, Sweden
Separating methods
A number of different separating methods have been considered in the report. The following table taken directly from the report summarized the findings:
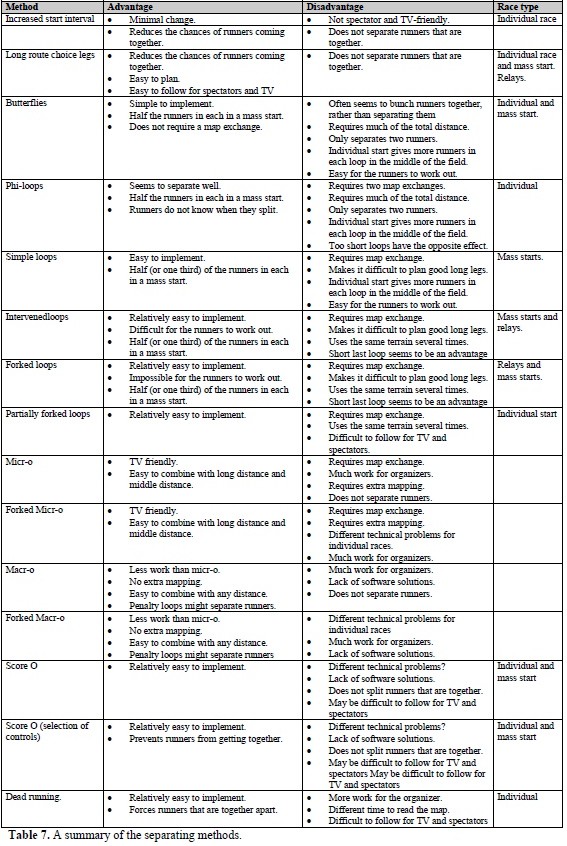
Regarding use of the separating methods for a WOC long distance with individual start (or similar event), the following conclusion is given in the report:
- The state-of-the-art methods to avoid grouping during the last years are butterflies and the last years also phi-loops. In addition course planners have had some focus on optimizing course characteristics. The other separating methods discussed in section 6 [Editors comment: given in the table above] do currently not seem to be able to replace butterflies / phi-loops for the WOC long distance.
In part I of this article series, the section in the report giving overall considerations that one should take when implementing phi-loops were given. In this article, I take a better look at phi-loops and how to implement them in practice.
Phi loops (taken directly from the report)
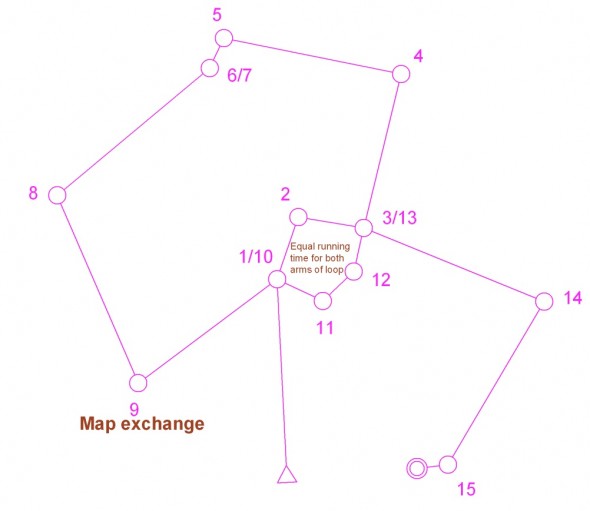
The loops are named after the Greek letter phi because they look somewhat like this letter [Editors comment: At least in its basic implementation]. There are two controls that are visited twice. At the first the runners are sent different ways through the phi-loop. At the second they are sent out in the common last part of the course. This method for separating the runners may involve one or two map exchanges in which case there is an additional “element of surprise” in the method (used at WOC 2007 long distance) – but may also be used without map exchange. For the case with map exchange(s), it requires more work for the organizers. As for butterflies it steals much of the total course length, and might make it more difficult to plan long, good route choice legs. The runners does not approach any control more than twice. The Ultuna-method is a variation of this with one (or more) controls along the diagonal as well [Editors comment: see illustration at the top of this article].
A special feature of the phi-loop method is the “element of surprise” which may be introduced by the fact that the runners can (in theory, but the course layout may tell something) never know if his/her next control is the same as another runner until after the map exchange. If more than one phi-loop is used in the course (i.e. a short second phi-loop towards the end of the course around the passing of a spectator control) and a course layout as shown to the right in Figure 18 is applied for the first part of the course, the “element of surprise” is extended to large parts of the course
This element of surprise may be introduced either by a normal phi-loop – or as a special variant of the Ultuna-method were the diagonal is outside of the phi-loop as shown to the left in figure 18. For the latter case, the “element of surprise” is extended to much larger parts of the course. The disadvantage is (1) potential unfairness due to some runners get the legs marked 1-3 early in the course – others late in the course and (2) you cannot compare split-times until after the phi-loops as runners have run different controls. Making running time for 1-3 and 10-13 as equal as possible – and also technical challenges and terrain as equal as possible, would address both these disadvantages.
On the other hand, if the running time for 1-3 and 10-13 differs by e.g. one fourth of the start interval (30 seconds for a 2 minute start interval), the probability for groups to form between control 3 and 10 would increase as effective start interval would be alternating between 1.30 and 2.30 – but the effectiveness of the spreading for 10-13 would increase significantly as there would be a time difference of 30 seconds built in to this part of the course. For a 3 minute start interval, this time difference between the legs of the phi-loop could be increased to 1 minute. The disadvantage here would be that you cannot compare split-times until after control 13. You could, however, in theory use extensive test-running to pre-calculate a time to be subtracted/added to the official splits published.
One advantage of phi loops compared to butterflies is that with phi-loops you reduce sharp angles – and thus it is more difficult to see other runners when approaching a control.
Phi loops: Implementation
Map from WOC 2007 in Ukraine, the only WOC where Phi-loops have been implemented.
In the following I take a look at how a good phi-loop spreading method could be implemented in practice. Here I depart from the report, which does not discuss practical implementation of phi-loops in detail.
In my opinion, a good phi-loop spreading method should always include at least one map exchange (like in WOC 2007 in Ukraine).
- The main argument against it is that it is more complicated for the organizers. This is a valid argument for smaller events, but not for large events like e.g. a World Ranking events, National Championships, or the World Orienteering Champs.
- The main argument for it is “the element of surprise”. I have had discussions with people claiming that this “element of surprise” is not important for top elite orienteers as they are excellent map readers. However, I think this element makes it more important for the runners to orienteer with highest accuracy in larger portions of the course, as they can not be 100% sure that they have the same control as other runners they meet in the forest.
Lets look at one example from the WOC 2009 Long distance map. This is not a perfect example, as I’ve not put the spreading method into two different parts of the course as in the figure at the very start of this article, but rather used the area of the butterfly from the WOC long distance course. Thus it is a lot easier to spot where the spreading occurs than what could have been possible with optimal placement of the spreading method. However, it is still instructive to compare this to the butterfly used at the championships. First we look at the butterfly used at WOC:
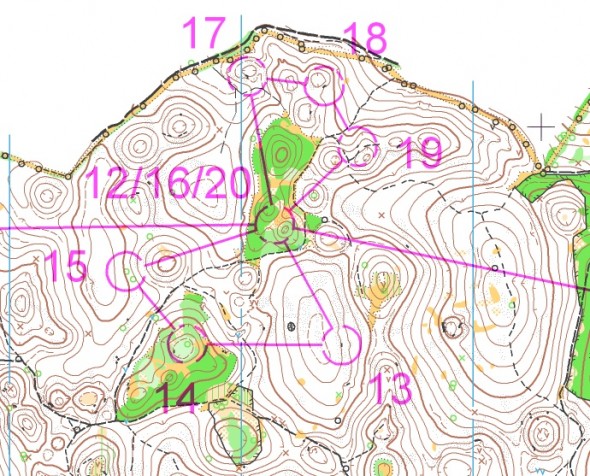
Then we look at one kind of phi-loop implementation (surely not the best, but still an example to let you see how the method can work). We start with the map the runner gets. Can you guess which of the controls which is different from the other runners? (Remember that the runner can not know if the different control was even earlier in the course. In theory it could have been already at the second control)
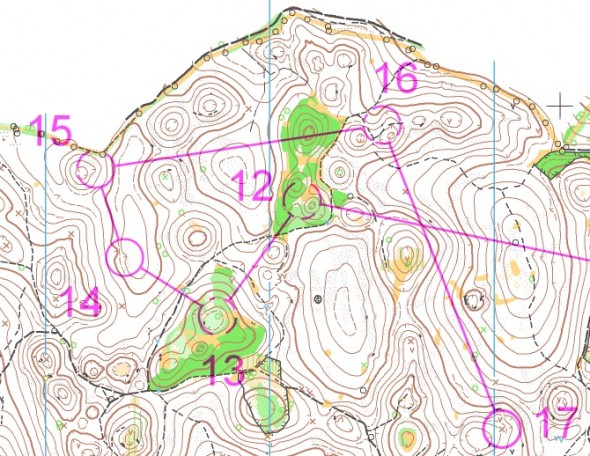
Then lets take a look at what the runner sees after the map exchange:
And finally the complete course in this area:
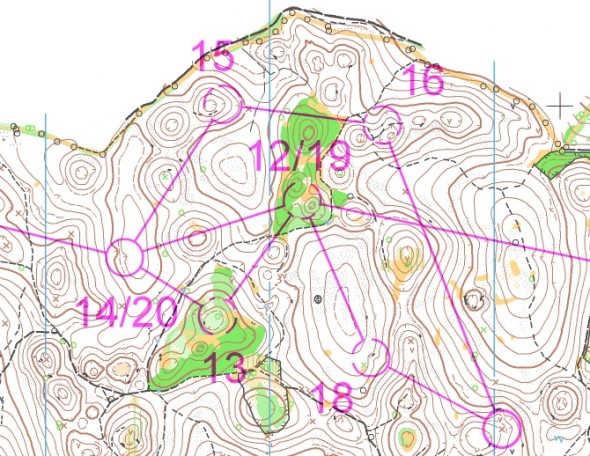
Note that I do not claim that this specific phi-loop implementation is better than the butterfly used in the WOC course – it is just an example of a phi-loop in a natural setting.
One interesting implementation of a phi-loop type separating method is this one suggested by Martin Lerjen – similar to WOC 2007 in Ukraine,
Have you got any comments about separating methods? Can you come up with a better example of a phi-loop? Have you examples of phi-loops which have worked well in competitions? Please add a comment below! Below you see one example – a phi-loop used at the start of a mass-start event in Mjølfjell, Norway (no map exchange),
